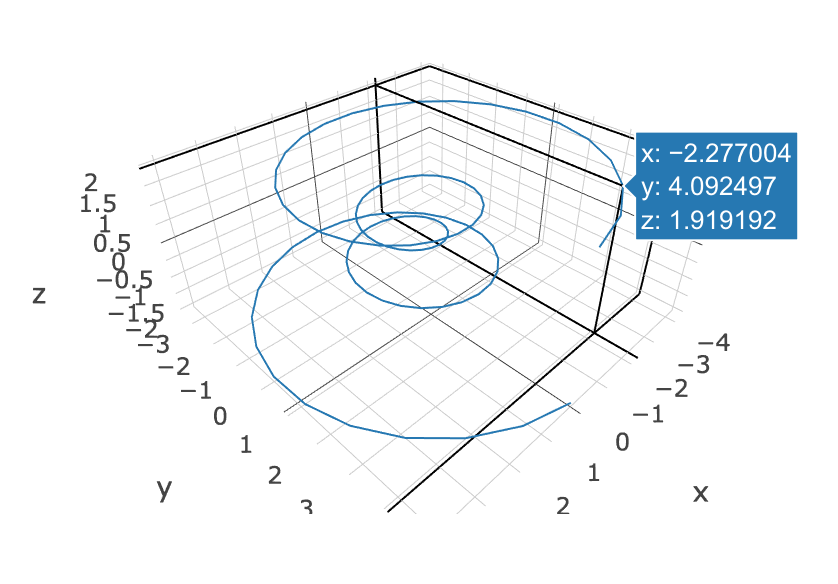
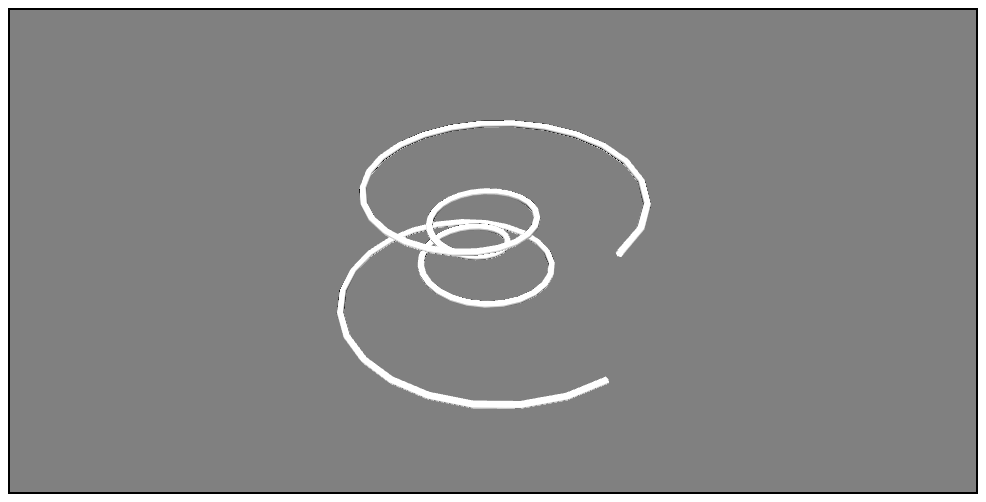
3次元の曲線をプロット
プロットするデータはこれ
Notebook
import numpy as np
theta = np.linspace(-4 * np.pi, 4 * np.pi, 100)
z = np.linspace(-2, 2, 100)
r = z**2 + 1
x = r * np.sin(theta)
y = r * np.cos(theta)
Matplotlib
Notebook
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import axes3d # <- 明示的には使わないが、インポートしておく必要がある。
# Notebook出力には次の１行が必要（%matplotlib inlineだとグラフを回転できない）
%matplotlib notebook
fig = plt.figure()
ax = fig.gca(projection='3d') # <- axes3dをインポートしていないとエラーになる。
ax.plot(x, y, z)
plt.show()
公式ドキュメント：[Line plots - mplot3d tutorial](http://matplotlib.org/mpl_toolkits/mplot3d/tutorial.html#line-plots)
Plotly
Notebook
from plotly.graph_objs import Scatter3d
from plotly.offline import init_notebook_mode, iplot
init_notebook_mode() # <- Notebook出力にはこの１行が必要
scatter = Scatter3d(x=x, y=y, z=z, mode='lines')
iplot([scatter]) # <- Notebookに出力するにはiplot関数を使う
公式ドキュメント：[3D Line Plots in Python](https://plot.ly/python/3d-line-plots/)
Mayavi
Notebook
from mayavi import mlab
mlab.init_notebook() # <- Notebook出力にはこの１行が必要
mlab.figure()
mlab.plot3d(x, y, z, tube_radius=0.1) # tube_radiusは線の太さ（省略可）
公式ドキュメント：[plot3d - Plotting functions](http://docs.enthought.com/mayavi/mayavi/auto/mlab_helper_functions.html#plot3d)
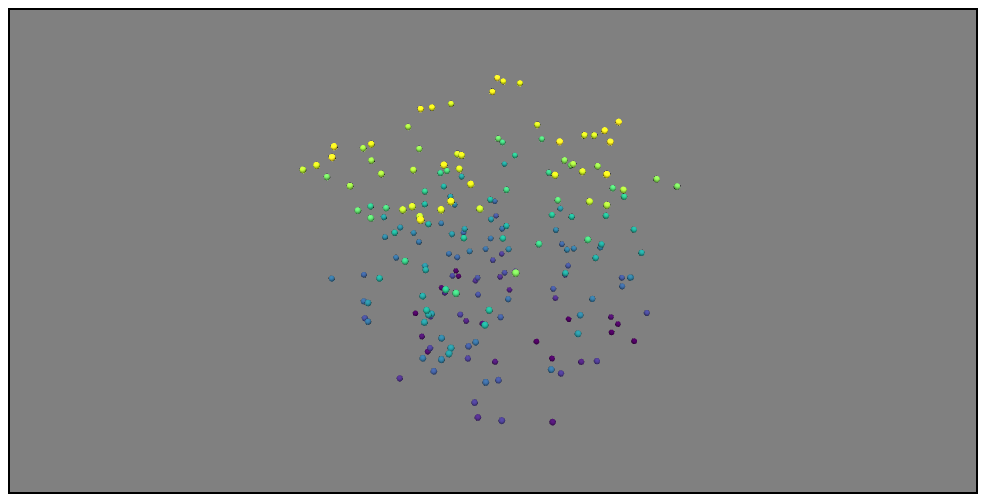
3次元の散布図をプロット
プロットするデータはこれ
Notebook
import numpy as np
X = np.random.uniform(-10, 10, 200)
Y = np.random.uniform(-10, 10, 200)
Z = np.random.uniform(-10, 10, 200)
Matplotlib
Notebook
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import axes3d # <- 明示的には使わないが、インポートしておく必要がある。
# Notebook出力には次の１行が必要（%matplotlib inlineだとグラフを回転できない）
%matplotlib notebook
fig = plt.figure()
ax = fig.gca(projection='3d') # <- axes3dをインポートしていないとエラーになる。
scatter = ax.scatter3D(X, Y, Z,
s=5, # マーカーのサイズ
c=Z, # 色分けに使う数値（任意の数値を指定可）
cmap=plt.cm.viridis) # 色のパターン
plt.colorbar(scatter) # カラーバーを表示（省略可）
plt.show()
公式ドキュメント：[Scatter plots - mplot3d tutorial](http://matplotlib.org/mpl_toolkits/mplot3d/tutorial.html#scatter-plots)
Plotly
Notebook
from plotly.graph_objs import Scatter3d
from plotly.offline import init_notebook_mode, iplot
init_notebook_mode() # <- Notebook出力にはこの１行が必要
# color、colorscale、showscaleは省略可
scatter = Scatter3d(x=X, y=Y, z=Z, mode='markers',
marker=dict(size=1, # マーカーのサイズ
color=Z, # 色分けに使う数値（任意の数値を指定可）
colorscale='Viridis', # 色のパターン
showscale=True) # カラーバーを表示
)
iplot([scatter]) # <- Notebookに出力するにはiplot関数を使う
公式ドキュメント：[3D Scatter Plots in Python](https://plot.ly/python/3d-scatter-plots/)
Mayavi
Notebook
from mayavi import mlab
mlab.init_notebook() # <- Notebook出力にはこの１行が必要
mlab.figure()
mlab.points3d(X, Y, Z,
Z, # 色分けに使う数値（任意の数値を指定可）
scale_mode='none', # マーカーを同じサイズで表示
scale_factor=0.5, # マーカーのサイズ
colormap='viridis') # 色のパターン
公式ドキュメント：[points3d - Plotting functions](http://docs.enthought.com/mayavi/mayavi/auto/mlab_helper_functions.html#points3d)
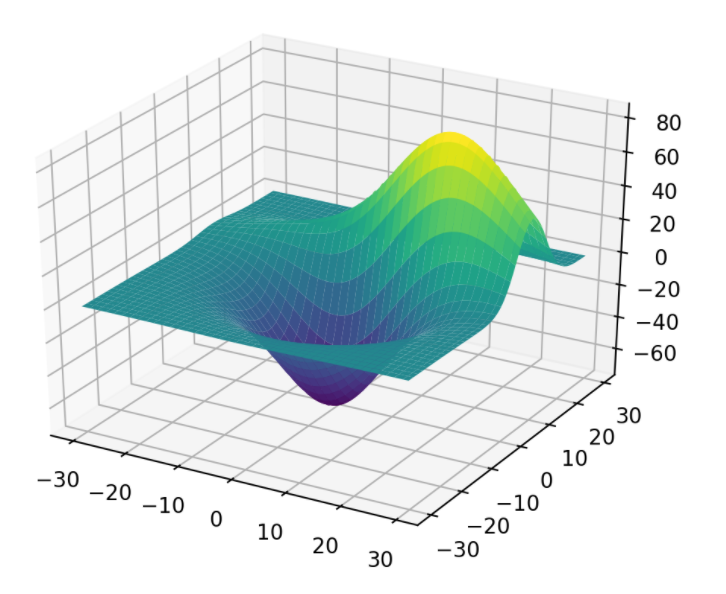
格子状に並んだ2.5次元データをプロット
プロットするデータはこれ
Notebook
from mpl_toolkits.mplot3d.axes3d import get_test_data
X, Y, Z = get_test_data()
Matplotlib
Notebook
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import axes3d # <- 明示的には使わないが、インポートしておく必要がある。
# Notebook出力には次の１行が必要（%matplotlib inlineだとグラフを回転できない）
%matplotlib notebook
fig = plt.figure()
ax = fig.gca(projection='3d') # <- axes3dをインポートしていないとエラーになる。
ax.plot_surface(X, Y, Z,
cmap=plt.cm.viridis) # 色のパターン（省略可）
plt.show()
公式ドキュメント：[Surface plots - mplot3d tutorial](http://matplotlib.org/mpl_toolkits/mplot3d/tutorial.html#surface-plots)
Plotly
Notebook
from plotly.graph_objs import Surface
from plotly.offline import init_notebook_mode, iplot
init_notebook_mode() # <- Notebook出力にはこの１行が必要
surface = Surface(x=X, y=Y, z=Z,
colorscale='Viridis') # 色のパターン（省略可）
iplot([surface]) # <- Notebookに出力するにはiplot関数を使う
公式ドキュメント：[3D Surface Plots in Python](https://plot.ly/python/3d-surface-plots/)
Mayavi
Notebook
from mayavi import mlab
mlab.init_notebook() # <- Notebook出力にはこの１行が必要
mlab.figure()
mlab.surf(Z,
colormap='viridis', # 色のパターン
warp_scale='auto') # Z軸のスケールを自動的に調整する
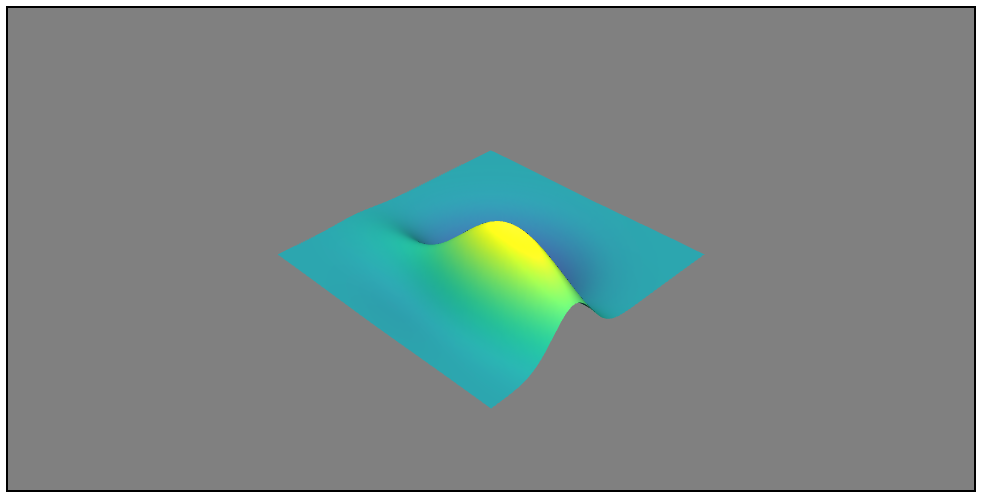公式ドキュメント：[surf - Plotting functions](http://docs.enthought.com/mayavi/mayavi/auto/mlab_helper_functions.html#surf)
不規則に並んだ2.5次元データをプロット
プロットするデータはこれ
Notebook
import numpy as np
X = np.random.uniform(-10, 10, 1000)
Y = np.random.uniform(-10, 10, 1000)
Z = X ** 2 + Y ** 2
Matplotlib
Notebook
import matplotlib.pyplot as plt
from mpl_toolkits.mplot3d import axes3d # <- 明示的には使わないが、インポートしておく必要がある。
# Notebook出力には次の１行が必要（%matplotlib inlineだとグラフを回転できない）
%matplotlib notebook
fig = plt.figure()
ax = fig.gca(projection='3d') # <- axes3dをインポートしていないとエラーになる。
ax.plot_trisurf(X, Y, Z, cmap=plt.cm.viridis) # cmapは色のパターン（省略可）
plt.show()
公式ドキュメント：[Tri-Surface plots - mplot3d tutorial](http://matplotlib.org/mpl_toolkits/mplot3d/tutorial.html#tri-surface-plots)
Plotly
Notebook
from plotly.graph_objs import Mesh3d
from plotly.offline import init_notebook_mode, iplot
init_notebook_mode() # <- Notebook出力にはこの１行が必要
mesh = Mesh3d(x=X, y=Y, z=Z,
delaunayaxis='z') # 三角形分割する時に投影する方向
iplot([mesh]) # <- Notebookに出力するにはiplot関数を使う
公式ドキュメント：[Surface Triangulation in Python](https://plot.ly/python/surface-triangulation/)
参考リンク
Jupyter notebookでMayaviを使う時の設定 - Qiita
Pythonの可視化パッケージの使い分け - Qiita
なんでもかんでもJupyter Notebookに表示するためのチートシート ２次元プロット編 - Qiita